Making Math Come to Life in a Grade 11 Workplace Class

Dear readers,

It has been a while since my last post. I'm sorry for the delay, but life got in the way! This post is dedicated to a fellow teacher candidate who goes above and beyond in her classroom. She is super smart, creative, and always comes up with phenomenal ideas for lessons and activities to use in the math classroom. As I mentioned in my previous posts, this semester is dedicated to learning about the different mathematics streams available from Grade 7-12. My classmate took on the Grade 11 Workplace stream and came up with a super awesome lesson that I cannot wait to try in my classroom-if I'm fortunate enough to teach Grade 11 Workplace that is!

As mathematics teachers, we constantly strive to find a way to make our units and lessons relevant for students; we need to make the math applicable to their lives, in order for them to want to learn. Otherwise, what is the point? We have questions like, "Why is this important? When will I ever need to use this? Why am I learning this?" A great way to answer these questions is to allow students to reflect and make connections between the math and real life. How could their learning be applied to the world? We know that reflection is the key to learning and higher order thinking, so we need to devote some time to student reflection.

In Grade 11 Workplace math, students are learning about budgeting and personal finance. Unfortunately, this can often be a boring topic for students, especially those who would rather spend their money, than save it. My candidate, Ms. T, designed an activity to allow students to learn about designing a budget, and planning an event, while giving them an opportunity to reflect on their choices and end result.



Ms. T wanted her students to plan a Friday night outing. She had two worksheets that the students could choose from: movies or bowling. When the students chose their outing for Friday night, they would work in pairs or groups of 3 to choose a budget for their night out. They would then figure out which movie theatre or bowling alley they would like to go to, while noting down the address in their workbooks. From there, the students will figure out how to get from their school to their choice of venue by choosing two modes of transportation and figuring out the cost of each. They will also calculate how much money it will cost them to buy a movie ticket or entry into the bowling alley; and how much they will spend on snacks or dinner. After they make each decision, they will reflect on why they made the choice they did. Finally, they will reflect on their overall budget: Did they stay within their budget or exceed their initial budget? What factors helped them stay within their budget or made them exceed their budget?



This was a really fun activity that is applicable to the students. They will all need to be able to plan and budget for a night out with their friends or family, so this activity allowed them to see the different aspects of their night that they need to account for, such as the destination, transportation, price of admission and additional foods and beverages. It also allows them to reflect on how they managed to stay within their budget or why they may have gone over their budget. I think that this activity will be something that they remember and use, not only when planning their Friday night, but when they are planning other trips and future events.

Mathematics Presentations Part 3: Grade 9 and 10 Applied

CBR

Welcome to another week of Math Presentations! This week in my Math Teaching course, I got a chance to see three more of my peers present on a lesson that they would use in either a grade 9 or 10 applied Mathematics class. My favourite presentation focused on a Grade 9 applied level class, learning about linear relations. This lesson could be used at the beginning of the linear relations unit, to introduce the slope of the line (rate of change) using a kinesthetic approach that students will love. The activity works by getting students into groups of 2-3. Each group receives a CBR, a calculator based ranger by Texas Instruments, along with a page of different graphs and their lines. A CBR is a calculator based ranger designed by Texas Instruments to track student motion using lines that will display on their TI calculators in a graph.

The goal of the activity is to have students try to replicate each graph by matching their motion to the slope of the lines in each graph. My group had a lot of fun figuring out which direction and speed to walk in order to match each given graph. By the end of the activity, we were able to understand the following:

1. Speed affects the slope of the line 

2. Walking fast produces a steep line 

3. Walking slow produces a fractional slope

4. Walking at a constant speed will produce a constant slope of 1. 

5. Walking away from the CBR will create a positive sloping line

6. Walking toward the CBR will create a negative sloping line 

I think that this activity would work really well in a grade 9 level applied classroom because it introduces the concept of linear relationships the students in a way that is fun and engaging. The students can literally graph their movement electronically to make their own linear path! They will be able to learn math, while getting some exercise in at the same time. I love this idea, and I would love to try it out in my own classroom! 

Math Presentations Part 2: Grade 9 Academic

Hi fellow bloggers! This week in my Math Teaching course was round 2 of math presentations. Four of my peers went up one by one to present on a math activity that they would do in a Grade 9 Math classroom. All of the presentations were excellent, and I found myself taking notes, so that I could try these in my Math placement. However, there is one activity in particular that I would like to focus on for today's blog. The topic was Grade 9 Geometry and the students would be learning about how to find the sum of the interior angles in any shape ranging from a triange (3 sided figure) to a myriagon (10,000 sides)!

In order to help students figure out an equation to solve for the sum of the interior angles, there was a guiding example that helped students make the connection! The teacher (Mr. F) would ask the class to draw any triangle that they wanted; it could be as small or as a big as they would like. The next step would be to figure out where the interior angles were on their triangles. When they found these as a class, he would instruct them to draw the angles in. They would then take a pair of scissors and cut out the triangle, before cutting out the interior angles. The key to this example is the interior angles. When the students have their 3 interior angles, they need to find a way to fit them together to make a semi-circle. Mr. F would guide them by asking what shape they just made; when they understood the shape, he would ask them to pull out their protractors and measure the angle of the semi-circle. It was 180 degrees! Even though each student drew a different triangle, they were still able to get the same result, which proves that the sum of the interior angles of any triangle will always equal 180 degrees. How cool!

Once the students had this knowledge, he passed out a guiding worksheet for the students to work on individually or in pairs. Even though it was an inquiry based worksheet that helped students to explore how to find the sum of the interior angles for any shape, it also provided some guidance to ground student thinking. It instructed students to try to make the smallest number of triangles from a single point A to any other vertex in the shape! I thought that this was a great way to keep students on track, yet keep it open enough so that they could experiment and try different things before coming to a solution. Finally, the students would have to fill in a chart to identify the relationship between the number of sides of a shape and the smallest number of triangles that they could draw inside of it. Overall, this was an outstanding activity!

I think that students from any stream (academic, applied, and possibly essential) could complete this activity because while it was geared towards students exploring and playing around with an abstract concept, it was also concrete in its guiding questions and semi-circle example. Students would be able to make the connections between the sum of the interior angles of a circle, the number of sides of a shape, and the number of triangles inside the shape. If students needed any more guidance, the teacher could provide one-on-one support, or more guiding questions, to help scaffold student learning. I will definitely try this in my class!

Math Presentations Part 1: Grade 7 or 8

Hi everyone! It's been a long time since my last blog post, but I am back for the new semester! Last week in my Math Teaching course, we were given the opportunity to present on a lesson activity that we would do in a grade 7 or 8 classroom. One of the presentations that really stood out to me was geared towards a Grade 8 level class learning about plotting coordinates (x, y) on a coordinate grid.

In order to spice up a traditionally dry mathematics concept, Ms. J decided to try something different. She took a kinesthetic approach to her activity by having students move around the classroom from station to station. She had eight stations set up around the classroom, so she divided the class into 8 groups and directed each group to a different station. At the first station, students would be given the coordinates for a stick figure's head and bottom. They would plot their stick figure on their coordinate grid, and they would fill in the accompanying chart to decide whether the stick figure was standing upright (head to bottom) or upside down (bottom to head). Once each group had figured out their man's initial position, they would be given a clue to help them find their next coordinate. Ms. F was helping them figure out transformations such as reflections and shifts, so each clue would focus on transforming Mr. Stick Figure's position in some way. When the students had completed their transformation and had the new coordinate position, they would need to look around the classroom to find the station that had their matching coordinate. At the next station, students would find their next transformation clue, complete the transformation, obtain the new coordinate, and move onto the station that had a matching coordinate. Students would proceed around the classroom like this until they had completed each of the eight stations.

As a reflection/consolidation of what they had learned, Ms. F would ask her students to take Mr. Stick Figure's current position, and help move him back home, so that he could go to sleep. In order to complete this task, students would need to know his last coordinate position, whether he was upright or upside down, and the coordinates of his house. They would need to find a transformation that would help get into a sleeping position (sideways) in his house!

I thought that this was a great activity because it got students moving and thinking, two things that are essential parts of learning. As teachers, we know that students cannot sit down for long periods of time because it's hard for them to sit still and concentrate; therefore, having them move around the classroom, while still doing math was a great way to promote student engagement, and give them an opportunity to stretch their limbs. I also loved the way that Ms. F helped students understand the transformations that were happening to Mr. Stick Figure because they could clearly see when he was shifting, reflecting, or moving from an upright position to an upside down position. If I ever get the opportunity to teach grade 8, I would love to try this in my classroom! However, I think that Ms. F also taught me that this lesson or concept of a scavenger hunt through the stations, can be applied in different grade levels and subjects across the curriculum. I am looking forward to incorporating movement and fun scavenger hunt activities in my math classroom!

Writing Scripts: Be prepared for all that the classroom throws at you!

Hi everyone!

Welcome to another weekly blog post from yours truly! Last week in my Math Teaching course, we learned about writing scripts for different parts of our lesson plan. Have you ever had an idea for a lesson activity or assignment, but felt unsure of how it would play out within the classroom? Would your students like the activity? Would it be too challenging or too easy? If it was too challenging, what guiding questions would you ask, in order to provide some clarity and understanding to your students? If it was too easy, how would you extend the activity to accommodate students who excel in this task?

As you look over your lesson plan for the day, you will be able to highlight the areas that you feel a little uncertain about implementing within the classroom. Speaking for myself, I know that I have trouble sleeping at night when I become anxious about not knowing how to handle the unexpected. In order to avoid the anxiety, I plan. I love planning because it gives me a sense of calm knowing that I am prepared for the unexpected. After I plan, I feel like I am ready to take on any challenge that is thrown my way because I have planned for it or something similar. Obviously, you are not able to plan for every single detail within your lesson because life is never that is easy. The beauty of teaching is that learning is spontaneous and each student's thought process and understanding is unique. Therefore, none of our lessons are going to turn out exactly the way we planned them, and that is great! It does make it easier on our nerves though, when we brainstorm possible scenarios that could arise during a particular activity or lesson, in order to create a general plan if students require additional support or extensions.

A few weeks ago, my partner and I created a math lesson plan that focused on Grade 9 Measurement in an applied level classroom. We designed a math center activity that allowed students to measure composite figures to find perimeter and area in a variety of different ways. One of our math center stations was tangrams (small shapes that make up a larger square). These tangrams can be used to create a number of different figures, such as a chair, boat, rocket, plane, etc.. We chose to have students work with a chair and a boat. They would need to use a ruler to measure the figure, in order to find the area and perimeter of their given composite shape. My partner and I realized that this
activity would be the most difficult for students to work with, so we decided to base our script off of this section of our lesson. Below is our script:

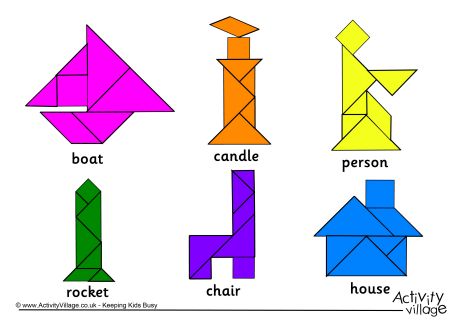Different Composite Figures
made from Tangrams

Lesson Plan Script

*see that group is struggling and has not started*

Teacher:  Hey guys, how’s it going?

Students murmur

Teacher: Do you guys know what you are looking for?

Student 1: Not really

Teacher: Okay, let’s look at the question and read it out loud. What is it asking you for?

Student 2:  We have to choose one of these shapes

Teacher: Perfect! Which one would you like to choose?

Student 1: I choose the boat!

Student 2: I kind of wanted to do the chair though…

Student 1: But boats are cool

Student 2: But the chair looks smaller

Student 1: I’ll do it if it’s easier

Teacher: Alright so we’re doing the chair. Now what do we need to do with the chair?

Students are silent in thought.

Teacher: Why don’t we look back at the question, and see what we need to do next?

Student 1: It says we need to find perimeter and area

Teacher: Okay. Which one would you like to work on first? Perimeter or area?

Student 2: Let’s do perimeter

Teacher: Alright, what information do we need to find perimeter?

Student 1: We need the side lengths. But there aren’t any lengths given.

Teacher: Well there is something provided at this station to help you measure that.

Student 2 grabs the rulers.

Teacher: Why don’t you guys get started and I’ll be back to check on you after I check on your classmates?

*10 minutes later: Teacher returns to check back on the group after observing the other groups*

Teacher observes that the students have measured all the side lengths of each smaller shape in the tangram and are trying to add them up.

Teacher:  Hi guys, how is it going?

Student 1: Much better! We have all the side lengths and now we are adding them together.

Teacher: Alright, well which sides did you include?

Student 2: All of them!

Teacher:  Let’s step back from the problem for a second and talk about perimeter. What do you think of when you hear the word perimeter?

Student 1: Well, the perimeter of a property.

Student 2: Or like the perimeter of a room.

Teacher: Then how would you describe perimeter in your own words?

Student 2: Well it’s kind of like when you go around the edge of something

Student 1: Yeah you like measure around a shape.

Teacher: Alright, and back to this activity, what shape did you choose?

Student 1: We chose a chair.

Teacher: Why don’t you guys sketch the chair for me?

Students roughly draw out similar chair outline. Teacher interrupts at this point.

Teacher: Alright, so this is your chair. How would you measure the perimeter of this?

Student 1 gestures around the outer edge: We would sum up these sides.

Student 2: But what about the other sides from the original picture?

Teacher: Well, are they a part of the perimeter you guys just showed me?

Student 2: Well, yes we need all the sides.

Student 1: But they aren’t actually outside sides. Only the ones in this picture are actually the outside edges of the chair.

Student 2: Why are they there then?

Teacher: Well tangrams are just a puzzle game that uses these standard small shapes to make new ones, like the chair and boat pictures. We aren’t using the tangrams right now as a puzzle, instead we are using their shapes to understand composite shapes, which are shapes made up of smaller shapes. So what measurements would you include in your perimeter for the chair?

Student 1 circles the edges of the tangram chair to show that he would measure the outside of the shape.

Student 2 circles the edges of the tangram as well.

Teacher: That’s right. So why don’t you use those sides to solve the perimeter, and then work on finding the total area of the chair?

-End of script-

I found that writing a script for this portion of the lesson was really helpful because it allowed me to think of where students would have the most trouble in deciphering what was being asked of them, and the steps that they would take to complete the task. By examining the word choice in the instructions for the activity, it gave me the opportunity to see where students might struggle and construct some guiding questions to provide clarity. I think that I would use this "script writing" method when planning most of my lessons because it made me feel more relaxed and confident in my teaching ability when I was able to put myself in the students' perspective and examine the activity.

I hope you all found this helpful! Enjoy the rest of your day!

Sincerely,
Dayna

Who doesn't love a good math game?

Hi everyone!

Welcome to another math post! This week in lecture, my classmates and I got the opportunity to play with some interactive math games- both online and offline.

The first game that we played is called Hedbanz. This is a classic game that people play at family events, board game nights with friends, and office parties (I imagine)! However, my professor altered it to help students practice their knowledge of the different forms of quadratic equations.

At the beginning of class, my professor handed out the headbands to every student in the classroom. Each headband had a quadratic equation on it, written in factor form, vertex form, and standard form. We were not allowed to look at the equation on our own headband. The goal of the game was to pair up with a partner, and ask each other "yes" or "no" questions, in order to figure out the equation on our forehead.

My best friend (K) and I immediately paired together to tackle this challenge. We put on our headbands and quickly wrote down some questions before we approached each other:

1. Am I in standard form? No
2. Am I in factored form? Yes
3. Do I have a coefficient in front of my brackets? No
4. Do I have more than one odd root? No
5. Do I have an odd root? No
6. Do I have two even roots? Yes
7. Do I have one positive, and one negative root? Yes
8. Are my roots the same digit? No
9. The only thing left to guess were the digits of our roots.

Results: K won the match, but we both had a great time and shared a few laughs during the game!

Here is a cute picture of the two of us afterwards! (Please forgive my awful selfie skills):

Why did my teacher let us play Hedbanz instead of handing out a worksheet on the different forms of quadratic equations?

Simple. It all comes down to three words that ALL teachers love: engagement, interactivity, and creativity.

My professor could have easily given us a worksheet, so that we could practice identifying the different forms of equations, but it would not have been nearly as successful! Even though the Hedbanz game was not a typical mathematics game, it gave students the opportunity to use math terminology in their questions (roots, standard, factored, vertex form, coefficient, etc.), and there was no pressure to arrive at a quick solution. Each pair could ask as many or as little questions as they needed to figure out their equation, and they were able to create questions that would help them best understand the different aspects of their equation.

This game is a great way to assess how well the students can use prior knowledge, problem solving and reasoning to figure out their headband equation. This game could be used as a diagnostic assessment before starting a unit on quadratic equations, or it could be used as a formative assessment before a major assignment or quiz. As the teacher observes the game unfold, he/she could make notes on areas of strengths and improvement for the class.

How can we use this game in our classroom? 

Hedbanz is versatile game that can be used to practice/review a number of math concepts in a fun and engaging way for students! We used it to test our knowledge of quadratic equations, but it can also be used to test students knowledge/application/thinking/communication skills in regards to prime factors,  graphs of functions, exponents, factoring, and more! The possibilities are endless for this game. This is also a great for ELL (English Language Learners) because it gives them an opportunity to practice asking questions, using math terminology, and demonstrating their knowledge by applying it in a low-risk setting that removes some of the anxiety and pressure from participating in a larger group setting.

I hope that you all enjoy this wonderful game, and have a great week ahead!

Dayna

Which one doesn't belong?

Hi everyone!

So today I thought we could play a math game! It's called "Which one doesn't belong?"

Objective:

There are four shapes/numbers/letters/graphs shown below; find a reason why each of the four (numbers) does not belong with the other three.

Rules: 

1. Have fun!

2. Be creative!

3. Try to think outside of the box.

4. Your thinking does not always have to be related to math!

------------------------------------------------------------------------------------------------

Take a moment, and try to come up with your own guesses/rules for why 9 does not belong with 16, 25, and 43.

My thoughts: 

The digits that make up 16, 25 and 43 all add up to 7. (16=1+6=7; 25=2+5=7; 43=4+3=7). The digits of 9 do not equal 7, so it does not belong. 

My mom's thoughts:

9 is the only single digit number! 

Both answers are right because they both find a rule that groups 16, 25, and 43 together such that 9 does not belong in the group. Both answers are mathematical because they use math terminology such as "add", "number", "single", "digit(s)". And both answers discuss the different properties of numbers.

In my math teaching course, we were introduced to a website called "Which one doesn't belong?" (www.wodb.ca). It was created by a woman named Mary Bourassa who accepts entries, tests them to see if they fit the criteria, and then posts them on her website for people to solve.

I love this activity because of it's flexibility. This activity presents a puzzle for students to solve, using their prior knowledge and experiences to help them find patterns, create categories/groups, and form rules that each number must follow in order to be part of the group. It is also OPEN to possibility. As long as a student sees a pattern that can be proven, their answer is correct! This opens up the playing field for all students because there is no "right answer" or set formula that has to be followed to arrive at an answer. This game is about being creative, open-minded, and thinking outside of the box.

I tested this game out with my mom because I wanted to see if we would get any different answers by examining the problem from different perspectives. My mom used to be a banker, so she is very good with numbers, but she doesn't study math as a hobby. This offered a new perspective because she thought in terms of the operations (addition, subtraction, multiplication, etc.) between numbers, while I tried to find patterns between the digits of a number. We tried three different "Which one doesn't belong?" puzzles, and I found that sometimes we came up with the same rule or category for a group, while other times, we had completely different responses! I think this would be a great activity to do with a class as a warm up exercise because it allows the teacher to perform a quick diagnostic assessment to see where students are at in their learning, but it is also a great opportunity for students to learn from each other and see that a puzzle can have multiple solutions.

As an expansion, I thought that it might be a fun challenge for students to try to make their own "Which one doesn't belong?" puzzle, and either share it with their classmates to solve, or e-mail it to Mary Bourassa. If it is solvable and Ms. Bourassa is able to test it successfully, then it may be published on her website for other people from around the world to solve. How cool would that be?

That's it for now! I hope everyone has a great weekend!

Dayna